Chapter 3: Hypernotes
WWW
3:1
TH Huxley, born 4th May 1825 and died 29th June 1895. Desmond, 1994
On Saturday 30th June 1860, at the British Association meeting in Oxford, in front of a crowd of 700 people, Huxley had effectively destroyed Samuel Wilberforce, the Bishop of Oxford with the celebrated put-down which has become an iconic moment for modern science:
“If ... the question is put to me would I rather have a miserable ape for a grandfather or a man highly endowed by nature and possessed of great means of influence ... who employs these faculties ... for the mere purpose of introducing ridicule into a grave scientific discussion, I unhesitatingly affirm my preference for the ape” (9578).
In The long revolution (1961), Raymond Williams says that “Huxley was a public educator, in the full sense...” – that is, he provided education rather than mere instruction. Huxley did recognise the dangers of attempting to popularise science:
“it must be admitted that the popularisation of science, whether by lecture or essay, has its drawbacks. Success in this department has its perils for those who succeed. The ‘people who fail’ take their revenge ... by ignoring all the rest of a man’s work and glibly labelling him a mere populariser.”
Huxley’s educational philosophy saw a key role for lectures (“[their] object is, in the first place, to awaken the attention and excite the enthusiasm of the student”). The content was also crucial, “commenc[ing] with the familiar facts of the scholar's daily experience”, the lecturer “should lead the beginner, step by step, to remoter objects and to the less readily comprehensible relations of things.” But it was not only content that made the lectures and books so popular. It was also the elegant, witty, seemingly effortless prose, the emphasis upon argument and process rather than mere description, and the fact that it was being done by one of the very great scientists of the age himself. The words were far from the remote pronouncements so typically handed down from the ivory towers. Such fluency did not come easily: “I found that the task of putting the truths learned in the field , the laboratory and the museum, into language which, without bating a jot of scientific accuracy, shall be generally intelligible, taxed such scientific and literary faculty as I possessed to the uttermost...”.
A fine example of Huxley's popular lecturing style is found in his lecture entitled On a piece of chalk (Huxley, 1967), delivered to a group of working men at the meeting of the British Association in Norwich, in 1868. It begins by considering the chalk which lay beneath the city of Norwich itself, and soon encompassed a vast mass of geology, geography and biology. In an interesting link with the theme of the present book, it was that same meeting at Norwich at which Broca and Hughlings Jackson both read papers on the lateralisation of language in the brain (Critchley & Critchley, 1998 pp. 93-4).
WWW
3:2
The sub-title of Physiography was ‘An introduction to the study of nature’. The last lines of the book returns the reader whence they had started:
“... the spectacle of the ebb and flow of the tide, under London Bridge, from which we started, proves to be a symbol of the working of forces which extend from planet to planet, and from star to star, throughout the universe” (Huxley, 1877 p.377)
The book is available on the internet at ??????.
WWW
3:3
A brief search on the Internet for ‘Left Bank’ finds hardly a city in the world that does not seem to have a restaurant called ‘The Left Bank’, and there are also innumerable presses, book shops and other places of left-of-centre intellectual activity with the same name. Ultimately almost all can probably trace their pedigree to Henri Murger's La Vie de Bohème, which formed the basis for Puccini’s La Bohème.
WWW
3:4
Quite why Huxley was so far out on this topic is an interesting question. It certainly was not due to Huxley being unaware of Kant – and in fact he not only mentions Kant in the introduction to the Physiography, but had also in January 1870 published a letter in Nature on Kant’s ideas about the nature of space. Neither is it because he could not read German – he had been reading German since childhood, took German philosophical works with him on the Rattlesnake, and earned money later in life by translating scientific books from German. Two possibilities remain. One is that Kant’s 1768 comments on right and left were less well known in the late nineteenth century than they are today, but that seems unlikely given that Kant refers to the problems of left and right again in 1770, in 1783 and in 1786. The other possibility is simply that Huxley didn’t write the passage, and perhaps didn’t even read the passage very closely. Huxley’s capacity for overwork and taking on too many projects was legion. In his review in Nature in January 1878, Judd refers to the role that his friend, a Mr Rudler, had played as “editor”, and that “To this cause we may attribute the small number of inaccuracies in either fact or expression which a careful perusal of the work has revealed”. A charitable explanation therefore is that this is simply not Huxley’s own work.
WWW
3:5
The method does require that midday is defined in a strict sense, when the sun is highest in the sky, and not the mid-day as indicated on clocks which nowadays are synchronised within different time-zones. Until the mid-nineteenth century this was the only meaning of noon everywhere, but the problems of co-ordinating railway timetables meant that our modern time-zones were created (Landes, 2000 pp.304-5).
WWW
3:6
Although the reversals were first proposed in 1906 by Benard Brunhes, they were first properly described in the 1950s when it was found that rock on the ocean floor had alternating bands of iron oxides pointed north and south. The magnetic field seems to have reversed about 170 times over the past 100 million years – about every 600,000 years; see Lowrie & Alvarez, 1981. The next reversal is predicted within the next two thousand years or so. (geography.about.com/education/scilife/ geography/library/weekly/aa032299.htm; www.amsci.org/amsci/articles/96articles/Fuller.html).
As well as the Earth's magnetic core shifting, it is also possible that the entire planet has become unstable and undergone major shifts in position, with the North Pole at times ending up at the equator (Irion, 2001).
WWW
3:7
The pattern of the stars in the night sky will change for at least two different reasons. Firstly, the earth is itself changing its position relative to the stars. As Hipparchus of Nicaea realised in the 2nd century BC, the positions of the stars at the equinoxes are therefore slightly different every year. This is now known as the ‘precession of the equinoxes’ and takes about 26,000 years to go through one full cycle. For its effect on the position of the Pole Star when the pyramids were being built, see Spence (2000a) Secondly, the stars are themselves moving (so-called, proper motion), as was first recognised by Sir James Halley (1656-1742) in 1718 when he compared the positions of the stars Sirius, Arcturus and Aldebaran with the positions reported by Ptolemy (died ca. 180 AD) in his Almagest. Although small, over eons these proper motions would result in the positions of the stars becoming increasingly different from their present positions.
WWW
3:8
Mullins (1999) concluded that no other planet in the Solar System was likely to provide a better home for the chap with the long white beard and the reindeers than did Earth. The recent appearance of open water at the North Pole as a result of global warming may mean that opinion needs to be revised.
For a discussion of why Venus is the only retrograde planet see Correia and Laskar (2001a).
WWW
3:9
There are complicated theories to do with the English in the 15th century buying port wine from Portugal, where they docked at O Porto, loading the red wine on the larboard side. www.whittsflying.com/Page5History.htm.
WWW
3:10
For the reader who wants a detailed analysis of almost anything to do with spirals and their nature, the best starting place is The curves of life (1914) by Sir Theodore Cook (1867-1928), which is still in print. A brief biographical account of Cook can be found in Kemp (1995).
The screw is only one example of the subtle and sophisticated engineering in everyday objects that we take for granted, objects such as the pencil, the subject of a superb biography by Henry Petroski (1989), or the table fork or the paper clip, also described by Petroski (1994). Rybczynski(2000b)'s account of the screw is in a similar vein, although a minor disappointment is that there is no discussion of when screws became right handed. Rybczynski has several engravings of early machines with wood screws, but they are extremely difficult to interpret because they may have been reversed during the printing process (e.g. the left-handed screw on p.60). The sole exception is the drawing on p.90, from c1475-1500, where it is clear that the wood screw in the lower half has a left-hand thread, and being a drawing the image is not reversed.
Any cyclist who has tried to take their bicycle as baggage on an international flight will have encountered left hand screws. Airlines like to pretend that they encourage cyclists, but in reality they invent a wealth of minor inconveniences which are designed to deter all but the most determined. One neat device, at check-in, is for them to say, “You must remove the pedals”. What the purpose of this is difficult to see – the bicycle hardly takes up any less room because of it. When asked to do remove the pedals, most people start by removing the right pedal, which is difficult enough since one usually doesn’t have the appropriate tools, and it has sometimes been tightened by cycling thousands of miles on it. But the real problem comes with the left pedal. One can strain and strain and nothing seems to happen. That is because it has a left hand thread, and turning in the conventional way is tightening not loosening it. Another nice trick by airlines is to say that the bike must be packed in a cardboard box “of the type available at any bicycle dealer”. Great; it sounds so easy. It isn’t much help, though, if one has just cycled over the Rockies or the Alps, and at midnight on a Saturday is trying to catch the flight home; cycling over mountains is not that easy, and even the keenest touring cyclists usually prefer not also to take a cardboard box bigger than the bike itself over the mountain passes.
Cook (1914 p.241-2) claims coffin screws, “that fortunately rare variety”, also had left-hand threads; I think I can vouch from my days as a hospital porter, where I was required to help undertakers remove bodies from the mortuary, that this did not apply in 1969. Cook also claims that “the screws of watches made in India [and other oriental countries]” had left-hand threads. I know of no evidence on this matter, although presumably there must be a definitive answer.
WWW
3:12
Ropes are described in terms of whether they have a clockwise twist, which is also known as a right-hand lay, or a ‘Z’ twist, or an anticlockwise, left-hand, or ‘S’ twist. Strictly, a single right-hand twist produces a yarn; several yarns are given a left-hand twist to form a strand; and several strands are given a right-hand twist when then are laid into a rope. If a rope has three strands it is known as a hawser, being hawser-laid. The top diagram below shows a right-hand twisted rope, with the left-hand twisted strands also visible. Cable-laid ropes can be either right or left-handed but are more usually left-hand, and are made by laying together three ropes, with a left-hand twist as in the lower diagram. Ropes only bed down properly if alternate layers are twisted to right and to left. When being coiled on the deck of a ship, right-hand ropes will only lie properly if coiled clockwise (‘with the sun’ as it is said), and left-hand ropes if coiled anti-clockwise. Interestingly, when plants such as honey-suckle grow over themselves, then the individual strands are left-handed, and the rope so formed is also left-handed (Cook, 1914). Of course unlike rope-making, the product is not being selected for its strength.
(Top) A right-hand lay rope consisting of three left-hand strands, each of which would be made up of right-hand lay yarns. (bottom). A left-hand lay cable comprised of three right-hand lay ropes.
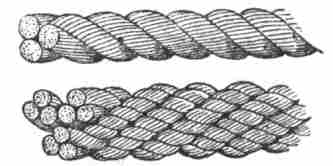
The convention for naming ropes has not always been as universal as it should have been. An anonymous pamphlet (Anonymous., 1878 p.12) described one expensive failure which resulted:
"The first Atlantic cable that was laid [in 1857] failed through a similar mistake. The Niagara's half was made in Liverpool, and the Agamemnon's half in London. It was only discovered, when too late, that the ropes were half right lay and half left. They tried to obviate the difficulty by covering the mid-ocean splice with a wooden shoot, or casing, but it only passed one message or so, and all that money was thrown into the sea...".
The reasons for the error are given in more detail elsewhere (Blake-Coleman, 1982 p.161). Ironically, one of the consultants on the exercise was the already distinguished physicist, William Thomson, later to be Lord Kelvin, who was subsequently the first to use the term 'chirality'.
The naming of right-hand spirals by botanists perhaps reflects the sort of twist a plant would make if it were growing upwards in the northern hemisphere and following the sun around which, as was seen in figure 2 of chapter 2, would mean a clockwise turn, and hence a left-hand spiral in the sense of left-hand screw thread.
I will not take the opportunity to quote the entire words of Flanders and Swann's Misalliance, although they would bear it. They can be found in Gardner (1990).
The twisting of thread is apparently also the basis of the distinction between jeans made by Levi Strauss and by Lee, Hugo Williams (2001b)telling us that,
"There is a gentleman's agreement among jean manufacturers that Levi's use a 'left-hand twill', while Lee jeans use a right. ... The toughest is left-hand for some reason; Levi's got first-choice because they were there first".
WWW
3:13
Cook (1914 pp.341-379) proposed that the rare form of Voluta vespertilio, seen in figure 3, was the model which Leonardo may have used to design the spiral staircase of the Château at Blois in the Loire.
WWW
3:14
Clark (1973) has pointed out that left and right differ from other place words. If we say “Mary is in front of the house” or “Mary is at the top of the house” the location is clearly relative to the house, whereas “Mary is to the right of the house” usually means to the right with respect to us, the viewer, and not from the house’s point of view.
An alternative method for actors to indicate right and left is to refer to 'prompt' and 'opposite prompt' (or 'off prompt'). It is a seemingly unambiguous system except that the prompter normally sits on the actors' left in the UK but the actors' right in the US. Corballis and Beale (1976 p.156) say that that French actors traditionally always had a courtyard, cour, to one side of the stage and a garden, jardin, to the other. Actors remembered which was which by punning cour, with coeur, the heart, on which side the courtyard would be found.
For an example of the disputes than can occur when proper right and proper left are not taken into account, see the article by Steven M. Wight (dobc.unipv.it/scrineum/scrineum.htm).
For reasons that I have never seen explained, and as Gardner (1990) has pointed out, striped men’s ties in Britain follow the pattern of the bend sinister, whereas those in America follow the pattern of the bend dexter. One presumes that this can have nothing to do with supposed legitimacy.
WWW
3:16
The river Pregel which flowed through Königsberg had two islands, which were connected to each other and the two banks by seven bridges. The question was whether it was possible walk over every bridge without recrossing any of them. The great Euler proved in 1735 that it was impossible.
A recent view of the old argument about the difference between the absolute and relative views of space, and physicists' arguments about the problems of how one can have acceleration without a frame to measure it, and hence the idea of an 'ether' which pervades all space, has been updated in quantum mechanics with the proposal that the vacumm itself has structure (see Davies (2001c).
WWW
3:18
Kant's collected works total 5651 pages, of which about half is critical apparatus.
I am not sure I know of any earlier reference than Kant which treats the universality of right-handedness as of crucial importance for understanding the nature of handedness and humanity, although there are undoubtedly earlier references which talk about right and left handedness (e.g. Plato and Aristotle).
The translation from Kant is taken from Van Cleve and Frederick (1991 p.30). There is an important distinction from the Cambridge edition (Walford & Meerbote, 1992) which says “all the peoples of the world are right-handed (apart from a few exceptions which ... do not upset the universality of the regular natural order)”. The implication in the latter is that there are some races, tribes or groups who do mostly write with the left hand. This distinction would be important in laterality research. Although the original German (Buchenau, 1922) is still somewhat ambiguous, it seems clear from the lines that follow that it is individual people who are the exceptions, rather than individual groups of people, as in the translation of Kerford and Walford (1968).
WWW
3:20
The railway track need not be straight as such, but it should not have any points used for changing tracks. It does not matter if the ends join up, in a circle, although the track should stay flat. It does not matter even if the track is on a Möbius strip, which is still one-dimensional.
WWW
3:21
In his brief comment, Wittgenstein pointed out that, “A right hand glove could be put on the left hand, if it could be turned round in four-dimensional space”. The philosopher Jonathan Bennett describes this remark as “mathematically sound but entirely unhelpful” (Bennett, 1970, reprinted in Van Cleve & Frederick, 1991 p.107).
In his The Plattner story, referred to elsewhere in this book, H G Wells puts very clearly the issue of rotation through a fourth dimension:
"There is no way of taking a man and moving him about in space, as ordinary people understand space, that will result in our changing his sides. Whatever you do, his right is still his right, his left his left. You can do that with a perfectly thin and flat thing, of course. If you were to cut a figure out of paper, any figure with a right and left side, you could change its sides simply by lifting it up and turning it over. But with a solid it is different. Mathematical theorists tell us that the only way in which the right and left sides of a solid body can be changed is by taking that body clean out of space as we know it,--taking it out of ordinary existence, that is, and turning it somewhere outside space. This is a little abstruse, no doubt, but any one with any knowledge of mathematical theory will assure the reader of its truth. To put the thing in technical language, the curious inversion of Plattner's right and left sides is proof that he has moved out of our space into what is called the Fourth Dimension, and that he has returned again to our world."
Philosophers object though that a mirror whilst producing the effect of rotating through a fourth dimension does not do it as a continuous transformation, unlike a rotation in a higher space. There are no intermediate positions which the mirror produces (one cannot stop halfway, for instance, unlike the case of picking up the triangle in figure 10).
WWW
3:23
HG Wells clearly understood the mathematical situation for in The Plattner Story he says, “There is no way of taking a man and moving him about in space, as ordinary people understand space, that will result in our changing his sides. Whatever you do, his right is still his right, his left his left. You can do that with a perfectly thin and flat thing, of course. If you were to cut a figure out of paper, any figure with a right and left side, you could change its sides simply by lifting it up and turning it over. But with a solid it is different.”
There are only three spatial dimensions at the macroscopic level at which our hands exist. If there are ten dimensions at a microscopic level, as superstring theories demand, that would have no consequence for Kant’s argument (Van Cleve, 1987).
A completely mirror-reversed person would starve to death, none of their enzymes being able to metabolise the L-amino acids and the D-sugars of which most food is composed. If the person were truly completely mirror-reversed they would also be made of anti-matter (Avalos et al., 1998 p.2393), rather than matter, with interestingly explosive consequences on their return.
There is an argument that in order to reject Kant’s argument it is not necessary to have a real fourth spatial dimension in which the object could be rotated. But that itself is a difficult argument, since if there were a fourth virtual dimension in which three-dimensional objects could be rotated, then as the philosopher James Van Cleve has pointed out, Kant would merely have had to consider instead not a three-dimensional hand but a four-dimensional ‘left’ hand. That could be rotated into four-dimensional ‘right’ hand by rotating it into a fifth dimension. But then Kant would merely have to propose a five-dimensional ‘left’ hand, to which the critic would invoke a sixth-dimensional rotation; and so on, for ever. The argument is what is called an ‘infinite regress’. However far it goes, the critic has problems with it, and Kant can always stay one step ahead (Van Cleve, 1987; reprinted in Van Cleve & Frederick, 1991 p.216.
WWW
3:24
A recent book devoted to Kant’s ideas on right and left, starts out, “Some ordinary facts about the world we live in can be readily explained by other ordinary facts. ... One ordinary fact that is not readily explainable in terms of other ordinary facts is the difference between left and right hands. ... [I]t is not obvious what facts about the world might explain the difference, or even, perhaps, what the difference is. Exactly what is it, after all, that makes a hand left and not right?” (Frederick, 1991).
Kant's different positions on the nature of space have meant that philosophers have tended to select those parts of Kant’s arguments that they find attractive. For instance, Bertrand Russell could claim, “these cases ... show, as Kant intended them to show, the essential relativity of space” (Russell, 1896).
One dominant position now in the philosophy of right and left is that Kant's original ideas were correct. The philosopher Graham Nerlich, in an article that “significantly changed the focus of the debate about Kant’s argument” (Frederick, 1991) acknowledges that “Kant himself had second and even third thoughts about his argument. My aim is to show that his first ideas were almost entirely correct about the whole of the issue” (Nerlich, 1973, reprinted in Van Cleve & Frederick, 1991).
WWW
3:25
Project Ozma was a search for radio messages from extra-terrestrials, started in 1960 at the Green Bank radio observatory in West Virginia. Frank Drake, who created the name, based it on the ‘humanoid’ ruler in the Oz books. (see Gardner, Gardner, 1990). Although Gardner does not acknowledge him, Borel (1960) seems to have presented the essential problem earlier, and done it in the context of communicating in Morse code with an extra-terrestrial on a cloud-covered planet, at a great distance, in an unknown direction, (Bennett, 1970, reprinted in Van Cleve & Frederick, 1991).
One of the founding fathers of modern psychology, William James (1842-1910), also made the same point about the impossibility of describing left and right using verbal labels, and of the need for direct comparison:
“If we take a cube and label one side top, another bottom, a third front, and a fourth back, there remains no form of words by which we can describe to another person which of the remaining sides is right and which left. We can only point and say here is right and there is left, just as we should say this is red and that blue” (James, 1890vol II, p.150).
Richard Feynman also presented the problem in the lectures he was giving at Caltech in about 1960, and which were published in 1963 (Feynman, Leighton, & Sands, 1963 p.52-8).Typically though he added several layers of physical sophisitication on top of it. Feynman is trying to tell the Martian how to build a model of a human being. Height can be specified in universals, such as “we are 17,000,000,000 hydrogen atoms high”, but the problem arises when the Martian is told, “ 'Now put the heart on the left side'. He says, 'Duhhh — the left side?'”. In typical Feynman fashion the story continues with the insight that the Martian, despite having left and right specified in terms of cobalt-60, could still get everything back to front if Martians happened to be made of anti-matter. An explosive end is possible, the fantasy continuing,
“What would happen when, after much conversation back and forth, we each have taught the other to make space ships and we meet halfway in empty space? We have instructed each other on our traditions, and so forth, and the two of us come rushing out to shake hands. Well, if he puts out his left hand, watch out!” (p.52-11).
Anonymous. 1878, Right and left hand screw propellors: Some practical hints by an old ship master, Cardiff: H.Morgan.
Avalos, M., Babiano, R., Cintas, P., Jiménez, J. L., Palacios, J. C., & Barron, L. D. 1998, "Absolute asymmetric synthesis under physical fields: Facts and fictions", Chemical Reviews, 98, 2391-2404.
Bennett, J. 1970, "The difference between right and left", American Philosophical Quarterly, 7 , 175-191.
Blake-Coleman, B. C. 1982, "The left heresy and directional preference in early science and technology", Folklore, 93, 151-163.
Borel, E. 1960, Space and Time, New York:
Buchenau, A. 1922, Vorkritische Schriften von Immanuel Kant, Band II, Berlin: Bruno Cassirer.
Clark, H. H. 1973, "Space, time, semantics, and the child," pp. 27-63 in Cognitive development and the acquisition of language, T. E. Moore, ed., New York: Academic Press.
Cook, T. A. 1914, The curves of life, Constable (Reprinted in Dover Books, 1979): London.
Corballis, M. C. & Beale, I. L. 1976, The psychology of left and right, Hillsdale, NJ: Lawrence Erlbaum Associates.
Correia, A. C. M. & Laskar, J. 2001a, "The four final rotation states of Venus", Nature, 411, 767-780.
Critchley, M. & Critchley, E. A. 1998, John Hughlings Jackson: Father of English neurology, Oxford: Oxford University Press.
Davies, P. 2001c, "Liquid space", New Scientist, 3rd November, 30-34.
Desmond, A. 1994, Huxley: The devil's disciple, London: Michael Joseph.
Feynman, R. P., Leighton, R. B., & Sands, M. 1963, The Feynman lectures on physics. Vol I: Mainly mechanics, radiation, and heat, Reading, MA: Addison-Wesley.
Frederick, R. E. 1991, "Introduction to the argument of 1768," pp. 1-14 in The philosophy of right and left, J. Van Cleve & R. E. Frederick, eds., Dordrecht: Kluwer.
Gardner, M. 1990, The new ambidextrous universe: revised edition, New York: W H Freeman.
Huxley, T. H. 1877, Physiography: An introduction to the study of nature, London: MacMillan.
Huxley, T. H. 1967, On a piece of chalk [edited by L. Eiseley], New York: Charles Scribner's Sons.
Irion, R. 2001, "Slip-sliding away", New Scientist, 18th August, 35-37.
James, W. 1890, Principles of Psychology, London: Macmillan.
Kemp, M. 1995, "Spirals of Life: D'Arcy Thompson and Theodore Cook, with Leonardo and Durer in Retrospect", Physis, 32, 37-54.
Kerford, G. B. & Walford, D. E. 1968, Kant: Selected pre-critical writings and correspondence with Beck, Manchester: Manchester University Press.
Landes, D. S. 2000, Revolution in time: Clocks and the making of the modern world, London: Viking.
Lowrie, W. & Alvarez, W. 1981, "One hundred million years of geomagnetic polarity history", Geology, 9, 392-397.
Mullins, J. 1999, "Grottoes new", New Scientist, 25th Dec 1999, 66-67.
Nerlich, G. 1973, "Hands, knees, and absolute space", Journal of Philosophy, 70, 337-351.
Petroski, H. 1989, The pencil, London: Faber and Faber.
Petroski, H. 1994, The evolution of useful things, New York: Vintage Books.
Russell, B. A. W. 1896, "The logic of geometry", Mind, 5, 23.
Rybczynski, W. 2000b, One good turn: A natural history of the screwdriver and the screw, New York: Simon and Schuster.
Spence, K. 2000a, "Ancient Egyptian chronology and the astronomical orientation of pyramids", Nature, 408, 320-324.
Van Cleve, J. 1987, "Right, left, and the fourth dimension", The Philosophical Review, 96, 33-68.
Van Cleve, J. & Frederick, R. E. 1991, The philosophy of right and left: Incongruent counterparts and the nature of space, Dordrecht: Kluwer.
Walford, D. & Meerbote, R. 1992, The Cambridge edition of the works of Immanuel Kant: Theoretical philosophy, 1755-1770, Cambridge: Cambridge University Press.
Williams, H. 2001b, "Freelance", Times Literary Supplement, December 28th, 14-14.
Williams, R. 1961, The long revolution, London: Chatto and Windus.